Should you wish to pay homage to the great physicist James Clerk Maxwell, you wouldn’t lack for locales in which to do it. There’s a memorial marker in London’s Westminster Abbey, not far from Isaac Newton’s grave. A magnificent statue was recently installed in Edinburgh, near his birthplace. Or you can pay your respects at his final resting place near Castle Douglas, in southwestern Scotland, a short distance from his beloved ancestral estate. They’re fitting monuments to the person who developed the first unified theory of physics, who showed that electricity and magnetism are intimately connected.
But what these landmarks don’t reflect is the fact that, at the time of Maxwell’s death in 1879, his electromagnetic theory—which underpins so much of our modern technological world—was not yet on solid ground.
An extraordinary amount of information about the world—the basic rules by which light behaves, current flows, and magnetism functions—can be boiled down to four elegant equations. Today, these are known collectively as Maxwell’s equations, and they can be found in just about every introductory engineering and physics textbook.
It could be argued that these equations got their start 150 years ago this month, when Maxwell presented his theory uniting electricity and magnetism before the Royal Society of London, publishing a full report the next year, in 1865. It was this work that set the stage for all the great accomplishments in physics, telecommunications, and electrical engineering that were to follow.
But there was a long gap between the presentation and the utilization. The mathematical and conceptual underpinnings of Maxwell’s theory were so complicated and counterintuitive that his theory was largely neglected after it was first introduced.
It took nearly 25 years for a small group of physicists, themselves obsessed with the mysteries of electricity and magnetism, to put Maxwell’s theory on solid footing. They were the ones who gathered the experimental evidence needed to confirm that light is made up of electromagnetic waves. And they were the ones who gave his equations their present form. Without the Herculean efforts of this group of “Maxwellians,” so named by historian Bruce J. Hunt, of the University of Texas at Austin, it might have taken decades more before our modern conception of electricity and magnetism was widely adopted. And that would have delayed all the incredible science and technology that was to follow.
Four Golden Rules
Today, the relationship between electricity and magnetism, along with the wave nature of light and electromagnetic radiation in general, is encoded in the four “Maxwell’s equations” shown above. The equations can be written in different ways. Here,
J is the current density.
E and
B are the electric and magnetic fields, respectively. And there are two other fields, the displacement field
D and the magnetic field
H. These fields are related to
E and
B by constants that reflect the nature of the medium that the fields pass through (the values of these constants in vacuum can be combined to give the speed of light). The displacement field
D was one of Maxwell’s key contributions, and the last equation describes how both current and changing electric fields can give rise to magnetic fields. The symbols at the beginning of each equation are differential operators. These compactly encode calculus that involves vectors, quantities that have a directionality and thus
x,
y, and
z components. Maxwell’s original formulation of his electromagnetic theory contained 20 equations.
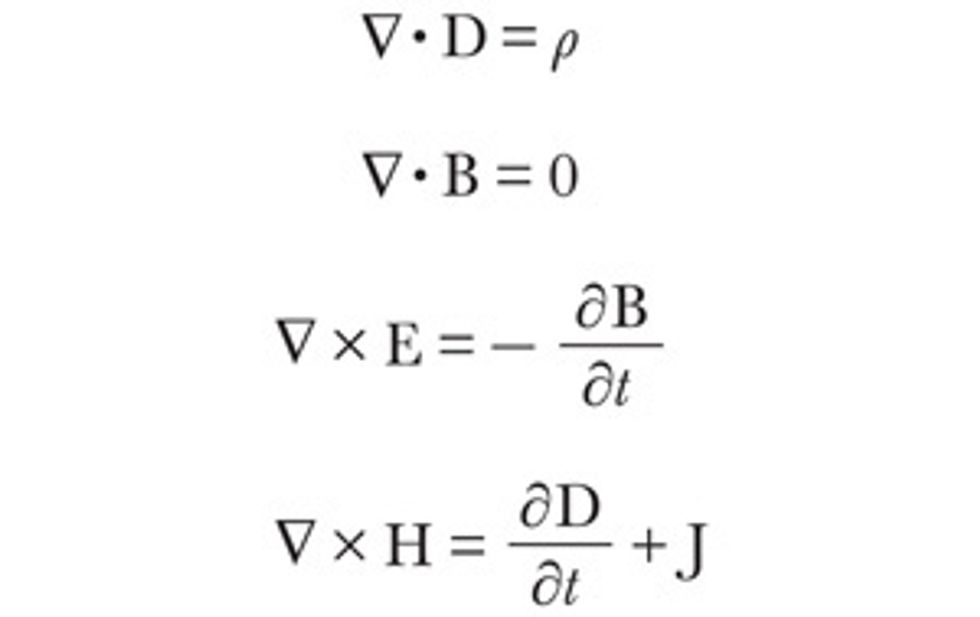
Today, we learn early on that visible light is just one chunk of the wide electromagnetic spectrum, whose radiation is made up of oscillating electric and magnetic fields. And we learn that electricity and magnetism are inextricably linked; a changing magnetic field creates an electric field, and current and changing electric fields give rise to magnetic fields.
We have Maxwell to thank for these basic insights. But they didn’t occur to him suddenly and out of nowhere. The evidence he needed arrived in bits and pieces, over the course of more than 50 years.
You could start the clock in 1800, when physicist Alessandro Volta reported the invention of a battery, which allowed experimenters to begin working with continuous direct current. Some 20 years later, Hans Christian Ørsted obtained the first evidence of a link between electricity and magnetism, by demonstrating that the needle of a compass would move when brought close to a current-carrying wire. Soon after, André-Marie Ampère showed that two parallel current-carrying wires could be made to exhibit a mutual attraction or repulsion depending on the relative direction of the currents. And by the early 1830s, Michael Faraday had shown that just as electricity could influence the behavior of a magnet, a magnet could affect electricity, when he showed that drawing a magnet through a loop of wire could generate current.
These observations were piecemeal evidence of behavior that no one really understood in a systematic or comprehensive way. What was electric current really? How did a current-carrying wire reach out and twist a magnet? And how did a moving magnet create current?
A major seed was planted by Faraday, who envisioned a mysterious, invisible “electrotonic state” surrounding the magnet—what we would today call a field. He posited that changes in this electrotonic state are what cause electromagnetic phenomena. And Faraday hypothesized that light itself was an electromagnetic wave. But shaping these ideas into a complete theory was beyond his mathematical abilities. That was the state of affairs when Maxwell came on the scene.
In the 1850s, after graduating from the University of Cambridge, in England, Maxwell set about trying to make mathematical sense of Faraday’s observations and theories. In his initial attempt, an 1855 paper called “On Faraday’s Lines of Force,” Maxwell devised a model by analogy, showing that equations that describe incompressible fluid flow could also be used to solve problems with unchanging electric or magnetic fields.
Maxwellian Milestones
-
1785
Charles-Augustin de Coulomb reports that the force between two charges varies with the inverse square of the distance.
-
1800
Alessandro Volta invents the first battery, which allowed experimenters to begin working with continuous direct current.
-
1820
Illustration: Wikipedia
Hans Christian Ørsted shows that the needle of a compass can move when brought close to a current-carrying wire, the first evidence of a link between electricity and magnetism.
Illustration: Wikipedia
His work was interrupted by a flurry of distractions. He took a job in 1856 at Marischal College, in Aberdeen, Scotland; devoted several years to a mathematical study of the stability of the rings of Saturn; was laid off in a college merger in 1860; and contracted smallpox and nearly died before finally taking a new job, as a professor at King’s College London.
Somehow, in all of this, Maxwell found the time to flesh out Faraday’s field theory. Although not yet a complete theory of electromagnetism, a paper he published in several parts in 1861 and 1862 proved to be an important stepping-stone.
Building on previous ideas, Maxwell envisioned a kind of molecular medium in which magnetic fields are arrays of spinning vortices. Each of these vortices is surrounded by small particles of some form that help carry spin from one vortex to another. Although he later laid it aside, Maxwell found that this mechanical vision helped describe a range of electromagnetic phenomena. Perhaps most crucially, it laid the groundwork for a new physical concept: the displacement current.
Displacement current isn’t really current. It’s a way of describing how the change in electric field passing through a particular area can give rise to a magnetic field, just as a current does. In Maxwell’s model, the displacement current arises when a change in electric field causes a momentary change in the position of the particles in the vortex medium. The movement of these particles generates a current.
One of the most dramatic manifestations of displacement current is in the capacitor, where in some circuits the energy stored between two plates in a capacitor oscillates between high and low values. In this system, it’s fairly easy to visualize how Maxwell’s mechanical model would work. If the capacitor contains an insulating, dielectric material, you can think of the displacement current as arising from the movement of electrons that are bound to the nuclei of atoms. These swing back and forth from one side to another, as if attached to stretched rubber bands. But Maxwell’s displacement current is more fundamental than that. It can arise in any medium, including the vacuum of space, where there are no electrons available to create a current. And just like a real current, it gives rise to a magnetic field.
With the addition of this concept, Maxwell had the basic elements he needed to link measurable circuit properties to two, now out-of-use, constants that express how readily electric and magnetic fields form in response to a voltage or a current. (Nowadays, we formulate these fundamental constants differently, as the permittivity and permeability of free space.)
Much as a spring constant determines how quickly a spring rebounds after it’s stretched or compressed, these constants can be combined to determine how fast an electromagnetic wave travels in free space. After others had determined their values using experiments on capacitors and inductors, Maxwell was able to estimate the speed of an electromagnetic wave in vacuum. When he compared the value to existing estimates of the speed of light, he concluded from their near equality that light must be an electromagnetic wave.
Maxwellian Milestones
-
1820
André-Marie Ampère shows that two parallel current-carrying wires can attract or repel each other, depending on the relative direction of their currents.
-
1831
Illustration: Wikipedia
Michael Faraday, who conceived of electrical and magnetic fields, discovers electromagnetic induction.
-
1831
Illustration: Universal History Archive/Getty Images
James Clerk Maxwell is born in Edinburgh.
Illustration: Wikipedia
Illustration: Universal History Archive/Getty Images
Maxwell completed the last key pieces of his electromagnetic theory in 1864, when he was 33 (although he made some simplifications in later work). In his 1864 talk and the paper that followed, he left the mechanical model behind but kept the concept of displacement current. Focusing on the mathematics, he described how electricity and magnetism are linked and how, once properly generated, they move in concert to make an electromagnetic wave.
This work is the foundation of our modern understanding of electromagnetism, and it provides physicists and engineers with all the tools they need to calculate the relationships among charges, electric fields, currents, and magnetic fields.
But what should have been a coup was actually met with extreme skepticism, even from Maxwell’s closest colleagues. One of the most vocal skeptics was Sir William Thomson (later Lord Kelvin). A leader of the British scientific community at the time, Thomson simply didn’t believe that such a thing as displacement current could exist.
His objection was a natural one. It was one thing to think of a displacement current in a dielectric filled with atoms. It was quite another to imagine it forming in the nothingness of a vacuum. Without a mechanical model to describe this environment and without actual moving electric charges, it wasn’t clear what displacement current was or how it might arise. This lack of a physical mechanism was distasteful to many physicists in the Victorian era. Today, of course, we’re willing to accept physical theories, such as quantum mechanics, that defy our everyday physical intuition, so long as they are mathematically rigorous and have great predictive power.
Maxwell’s contemporaries perceived other big shortcomings in his theory. For example, Maxwell postulated that oscillating electric and magnetic fields together form waves, but he didn’t describe how they move through space. All waves known at this time required a medium in which to travel. Sound waves travel in air and water. So if electromagnetic waves existed, physicists of the time reasoned, there must be a medium to carry them, even if that medium couldn’t be seen, tasted, or touched.
Maxwell, too, believed in such a medium, or ether. He expected that it filled all of space and that electromagnetic behavior was the result of stresses, strains, and movements in this ether. But in 1865, and in his later two-volume Treatise on Electricity and Magnetism, Maxwell presented his equations without any mechanical model to justify how or why these mystical electromagnetic waves could possibly propagate. For many of his contemporaries, this lack of a model made Maxwell’s theory seem grievously incomplete.
Perhaps most crucially, Maxwell’s own description of his theory was stunningly complicated. College students may greet the four Maxwell’s equations with terror, but Maxwell’s formulation was far messier. To write the equations economically, we need mathematics that wasn’t fully mature when Maxwell was conducting his work. Specifically, we need vector calculus, a way of compactly codifying the differential equations of vectors in three dimensions.
Maxwell’s theory today can be summed up by four equations. But his formulation took the form of 20 simultaneous equations, with 20 variables. The dimensional components of his equations (the x, y, and z directions) had to be spelled out separately. And he employed some counterintuitive variables. Today, we are accustomed to thinking of and working with electric and magnetic fields. But Maxwell worked primarily with another kind of field, a quantity he called electromagnetic momentum, from which he would then calculate the electric and magnetic fields that Faraday first envisioned. Maxwell may have selected that name for the field—today known as magnetic vector potential—because its derivative with respect to time yields an electric force. But the potential does us no favors when it comes to calculating a lot of simple electromagnetic behavior at boundaries, such as how electromagnetic waves reflect off a conductive surface.
The net result of all of this complexity is that when Maxwell’s theory made its debut, almost nobody was paying attention.
But a few people were. And one of them was Oliver Heaviside. Once described by a friend as a “first rate oddity,” Heaviside, who was raised in extreme poverty and was partially deaf, never attended university. Instead, he taught himself advanced science and mathematics.
Heaviside was in his early 20s and working as a telegrapher in Newcastle, in northeast England, when he obtained Maxwell’s 1873 Treatise. “I saw that it was great, greater and greatest,” he later wrote. “I was determined to master the book and set to work.” The next year, he left his job and moved in with his parents to learn Maxwell.
It was Heaviside, working largely in seclusion, who put Maxwell’s equations in their present form. In the summer of 1884, Heaviside was investigating how energy moved from place to place in an electrical circuit. Is that energy, he wondered, carried by the current in a wire or in the electromagnetic field surrounding it?
Heaviside ended up reproducing a result that had already been published by another British physicist, John Henry Poynting. But he kept pushing further, and in the process of working through the complicated vector calculus, he happened upon a way to reformulate Maxwell’s score of equations into the four we use today.
The key was eliminating Maxwell’s strange magnetic vector potential. “I never made any progress until I threw all the potentials overboard,” Heaviside later said. The new formulation instead placed the electric and magnetic fields front and center.
Maxwellian Milestones
-
1873
Maxwell publishes his magnum opus, A Treatise on Electricity and Magnetism, which contains further mathematical and interpretive work.
-
1879
Illustration: Popperfoto/ Getty Images
Heinrich Hertz’s interest in Maxwell’s work is piqued by a Prussian Academy of Sciences competition to obtain experimental evidence for or against electro-magnetic waves.
-
1879
Maxwell dies of stomach cancer at age 48.
Illustration: Popperfoto/ Getty Images
One of the consequences of the work was that it exposed the beautiful symmetry in Maxwell’s equations. One of the four equations describes how a changing magnetic field creates an electric field (Faraday’s discovery), and another describes how a changing electric field creates a magnetic field (the famous displacement current, added by Maxwell).
This formulation also exposed a mystery. Electric charges, such as electrons and ions, have lines of electric field around them that radiate from the charge. But there is no source of magnetic field lines: In our known universe, magnetic field lines are always continuous loops, with no start or end.
This asymmetry troubled Heaviside, so he added a term representing a magnetic “charge,” assuming that it had just not yet been discovered. And indeed it still hasn’t. Physicists have since conducted extensive searches for such magnetic charges, also called magnetic monopoles. But they have never been found.
Still, magnetic current is a useful artifice for solving electromagnetic problems with some kinds of geometries, such as the behavior of radiation moving through a slit in a conductive sheet.
If Heaviside modified Maxwell’s equations to this degree, why don’t we call them Heaviside’s equations? Heaviside answered this question himself in 1893 in the preface to the first volume of his three-volume publication, Electromagnetic Theory. He wrote that if we have good reason “to believe that he [Maxwell] would have admitted the necessity of change when pointed out to him, then I think the resulting modified theory may well be called Maxwell’s.”
Mathematical elegance was one thing. But finding experimental evidence for Maxwell’s theory was something else. When Maxwell passed away in 1879, at age 48, his theory was still considered incomplete. There was no empirical evidence that light is composed of electromagnetic waves, aside from the fact that the speed of visible light and that of electromagnetic radiation seemed to match up. In addition, Maxwell did not specifically address many of the qualities electromagnetic radiation should have if it makes up light, namely behaviors like reflection and refraction.
Physicists George Francis FitzGerald and Oliver Lodge worked to strengthen the link to light. Proponents of Maxwell’s 1873 Treatise, the pair met the year before Maxwell’s death at a meeting of the British Association for the Advancement of Science in Dublin, and they began collaborating, largely through the exchange of letters. Their correspondence with each other and with Heaviside helped to advance the theoretical understanding of Maxwell’s theory.
As historian Hunt outlines in his book, The Maxwellians, Lodge and FitzGerald also hoped to find experimental evidence to support the idea that light is an electromagnetic wave. But here they didn’t have much success. In the late 1870s, Lodge developed some circuitry that he hoped would be capable of converting lower-frequency electricity into higher-frequency light, but the effort fizzled when Lodge and FitzGerald realized their schemes would create radiation of too low a frequency to be detected by eye.
Maxwellian Milestones
-
1885
Illustration: SSPL/Getty Images
Oliver Heaviside publishes a condensed version of Maxwell’s equations, reducing the equation count from 20 to four.
-
1888
Hertz, several years after moving to a well-equipped laboratory in Karslruhe, reports confirmation of the existence of the electromagnetic waves predicted by Maxwell.
-
1940
Albert Einstein gives the term “Maxwell’s equations” a boost with his monograph “Considerations Concerning the Fundaments of Theoretical Physics.”
Illustration: SSPL/Getty Images
Nearly a decade later, Lodge was performing experiments on lightning protection when he noticed that discharging capacitors along wires produced arcs. Curious, he changed the wire lengths and found that he could realize spectacular sparks. He correctly deduced that this was the action of an electromagnetic wave in resonance. He found that with enough power, he actually could see the air becoming ionized around the wires, a dramatic illustration of a standing wave.
Now confident that he was generating and detecting electromagnetic waves, Lodge planned to report his astounding results at a meeting of the British Association, right after he returned from a vacation in the Alps. But while reading a journal on the train out of Liverpool, he discovered he’d been scooped. In the July 1888 issue of Annalen der Physik, he found an article entitled “Über elektrodynamische Wellen im Luftraum und deren Reflexion” (“On electrodynamic waves in air and their reflection”) written by a little-known German researcher, Heinrich Hertz.
Hertz’s experimental work on the subject began at the Technische Hochschule (now the Karlsruhe Institute of Technology) in Karlsruhe, Germany, in 1886. He noticed that something curious happened when he discharged a capacitor through a loop of wire. An identical loop a short distance away developed arcs across its unconnected terminals. Hertz recognized that the sparks in the unconnected loop were caused by the reception of electromagnetic waves that had been generated by the loop with the discharging capacitor.
Inspired, Hertz used sparks in such loops to detect unseen radio-frequency waves. He went on to conduct experiments to verify that electromagnetic waves exhibit lightlike behaviors of reflection, refraction, diffraction, and polarization. He performed a host of experiments both in free space and along wires. He molded a meter-long prism made of asphalt that was transparent to radio waves and used it to observe relatively large-scale examples of reflection and refraction. He launched radio waves toward a grid of parallel wires and showed that they would reflect or pass through the grid depending on the grid’s orientation. This demonstrated that electromagnetic waves were transverse: They oscillate, just as light does, in a direction perpendicular to the direction of their propagation. Hertz also reflected radio waves off a large sheet of zinc, measuring the distance between canceled-out nulls in the resulting standing waves in order to determine their wavelengths.
With this data—along with the frequency of the radiation, which he calculated by measuring the capacitance and inductance of his circuitlike transmitting antenna—Hertz was able to calculate the speed of his invisible waves, which was quite close to that known for visible light.
Radio Magic: Heinrich Hertz used the coil (left) and the antennas (right) to produce and detect electromagnetic radiation outside the visible range.
Photo: Karlsruhe Institute of Technology Archives
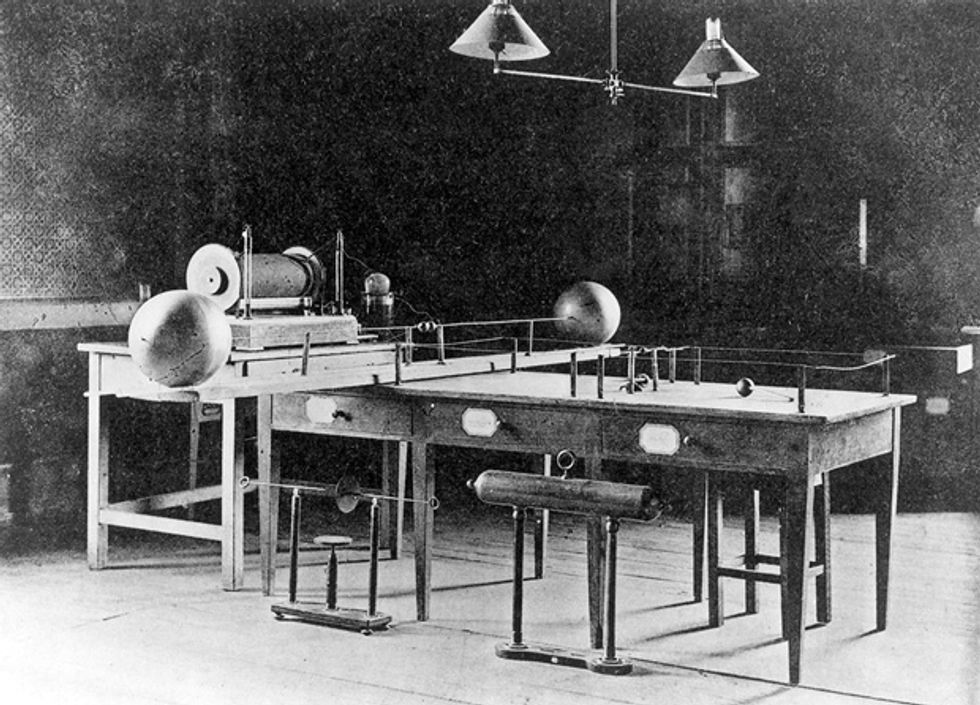
Maxwell had postulated that light was an electromagnetic wave. Hertz showed that there was likely an entire universe of invisible electromagnetic waves that behave just as visible light does and that move through space at the same speed. This revelation was enough, by inference, for many to accept that light itself is an electromagnetic wave.
Lodge’s disappointment at being scooped was more than compensated by the beauty and completeness of Hertz’s work. Lodge and FitzGerald worked to popularize Hertz’s findings, presenting them before the British Association. Almost immediately, Hertz’s work went on to inform the development of wireless telegraphy. The earliest incarnations of the technology employed transmitters much like the broadband spark-gap devices Hertz used.
Eventually scientists accepted that waves could travel through nothing at all. And the concept of a field, at first distasteful because it lacked any mechanical parts to make it work, became central to much of modern physics.
There was much more to come. But even before the close of the 19th century, thanks to the dogged efforts of a few dedicated enthusiasts, Maxwell’s legacy was secure.
About the Author
James C. Rautio is the founder of Sonnet Software.
Mike McQuade
