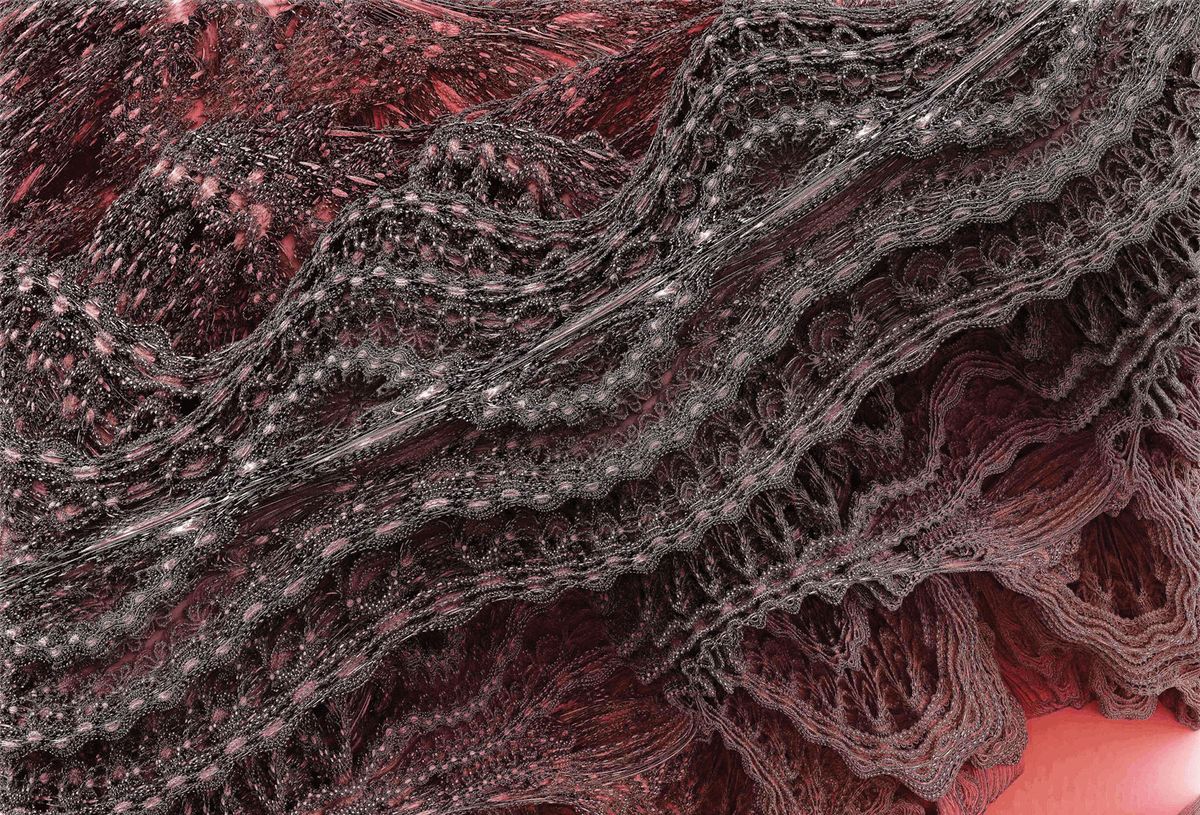
Who said algebra isn’t fun? This ornately detailed figure, which brings to mind the craggy but beautiful contours of a coral reef, is actually a 3-D computer rendering of a mathematical equation. It’s an example of a fractal, which breaks the rules of traditional geometry because its area and perimeter are incalculable. The numbers behind these beasties can’t be crunched, because no matter how closely you zoom in, the features always look like ever-smaller copies of the entire figure. Widely available fractal-generating software allows anyone to plot simple designs. This highly intricate figure, produced by Daniel White, a Web developer and amateur mathematician based in Bedford, England, is one of many at his Web site, https://www.skytopia.com.
This article appears in the March 2010 print issue as “Painting by Numbers.”