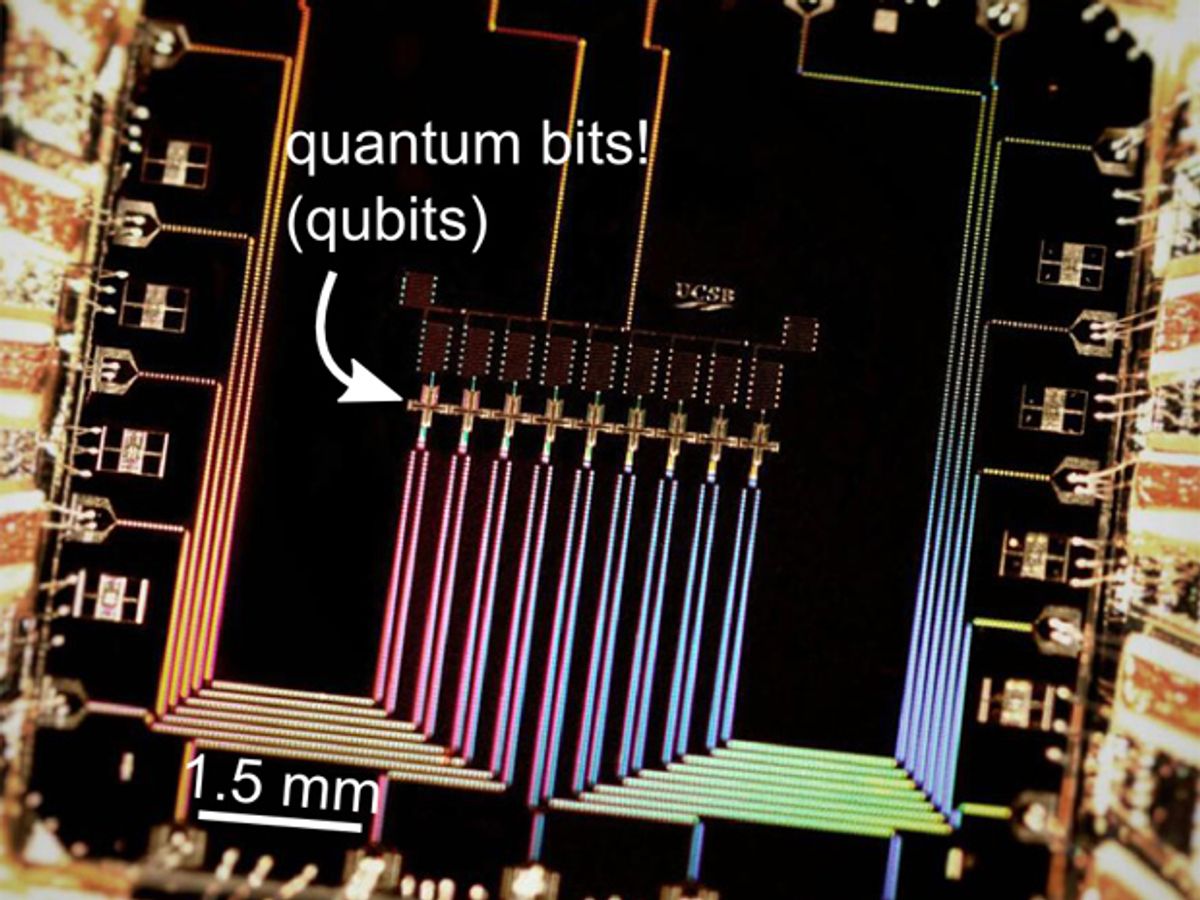
Quantum computers won’t ever outperform today’s classical computers unless they can correct for errors that disrupt the fragile quantum states of their qubits. A team at Google has taken the next huge step toward making quantum computing practical by demonstrating the first system capable of correcting such errors.
Google’s breakthrough originated with the hiring of a quantum computing research group from the University California, Santa Barbara in the autumn of 2014. The UCSB researchers had previously built a system of superconducting quantum circuits that performed with enough accuracy to make error correction a possibility. That earlier achievement paved the way for the researchers—many now employed at Google—to build a system that can correct the errors that naturally arise during quantum computing operations. Their work is detailed in the 4 March 2015 issue of the journal Nature.
“This is the first time natural errors arising from the qubit environment were corrected,” said Rami Barends, a quantum electronics engineer at Google. “It’s the first device that can correct its own errors.”
Quantum computers have the potential to perform many simultaneous calculations by relying upon quantum bits, or qubits, that can represent information as both 1 and 0 at the same time. That gives quantum computing a big edge over today’s classical computers that rely on bits that can only represent either 1 or 0.
But a huge challenge in building practical quantum computers involves preserving the fragile quantum states of qubits long enough to run calculations. The solution that Google and UCSB have demonstrated is a quantum error-correction code that uses simple classical processing to correct the errors that arise during quantum computing operations.
Such codes can’t directly detect errors in qubits without disrupting the fragile quantum states. But they get around that problem by relying on entanglement, a physics phenomenon that enables a single qubit to share its information with many other qubits through a quantum connection. The codes exploit entanglement with an architecture that includes “measurement” qubits entangled with neighboring “data” qubits.
The Google and UCSB team has been developing a specific quantum error-correction code called “surface code.” They eventually hope to build a 2-D surface code architecture based on a checkerboard arrangement of qubits, so that “white squares” would represent the data qubits that perform operations and “black squares” would represent measurement qubits that can detect errors in neighboring qubits.
For now, the researchers have been testing the surface code in a simplified “repetition code” architecture that involves a linear, 1-D array of qubits. Their unprecedented demonstration of error correction used a repetition code architecture that included nine qubits. They tested the repetition code through the equivalent of 90,000 test runs to gather the necessary statistics about its performance.
“This validates years and years of theory to show that error correction can be a practical possibility,” said Julian Kelly, a quantum electronics engineer at Google.
Just as importantly, the team’s demonstration showed that error correction rates actually improved when they increased the number of qubits in the system. That’s exciting news for quantum computing, because it shows that larger systems of qubits won’t necessarily collapse under a growing pile of errors. It means that larger quantum computing systems could be practical.
For instance, the team compared the error rate of a single physical qubit with the error rate of multiple qubits working together to perform logic operations. When they used the repetition code array of five qubits, the logical error rate was 2.7 times less than the single physical qubit error rate. A larger array of nine qubits showed even more improvement with a logical error rate 8.5 times less than the single physical qubit error rate. As Kelly explains:
“One, we wanted to show a system where qubits are cooperating and outperforming any single qubit. That’s an important milestone. Even more exciting than that, when we go from five to nine qubits, it gets better. If you want to get a better error rate, you add more qubits.”
This first demonstration of error correction shows a clear path forward for scaling up the size of quantum computing systems. But on its own terms, it still falls well short of the error correction rate needed to make quantum computing practical, said Austin Fowler, a quantum electronics engineer at Google. The team would need to improve error correction rates by 5 to 10 times in order to make quantum computing truly practical.
Still, the current quantum computing system managed to maintain coherence in the quantum states of its qubits despite all the uncorrected errors—a fact that left the researchers feeling optimistic about the future. Their work was supported with government funding from the Intelligence Advanced Research Projects Activity and Army Research Office grants.
The latest error correction demonstration would mainly work with universal logic-gate quantum computers; systems that would represent super-fast versions of today’s classical “gate-model” computers. But Google has also invested in the alternate “quantum annealing” approach of Canadian company D-Wave Systems.
D-Wave’s quantum annealing machines have sacrificed some qubit coherence to scale up quickly in size to the 512-qubit D-Wave Two machine—a system that dwarfs most experimental quantum computing systems containing just several qubits. Google has turned to John Martinis, a professor of physics at the University of California, Santa Barbara, along with his former UCSB researchers now on Google’s payroll, to try building a more stabilized version of D-Wave’s quantum annealing machines.
Jeremy Hsu has been working as a science and technology journalist in New York City since 2008. He has written on subjects as diverse as supercomputing and wearable electronics for IEEE Spectrum. When he’s not trying to wrap his head around the latest quantum computing news for Spectrum, he also contributes to a variety of publications such as Scientific American, Discover, Popular Science, and others. He is a graduate of New York University’s Science, Health & Environmental Reporting Program.